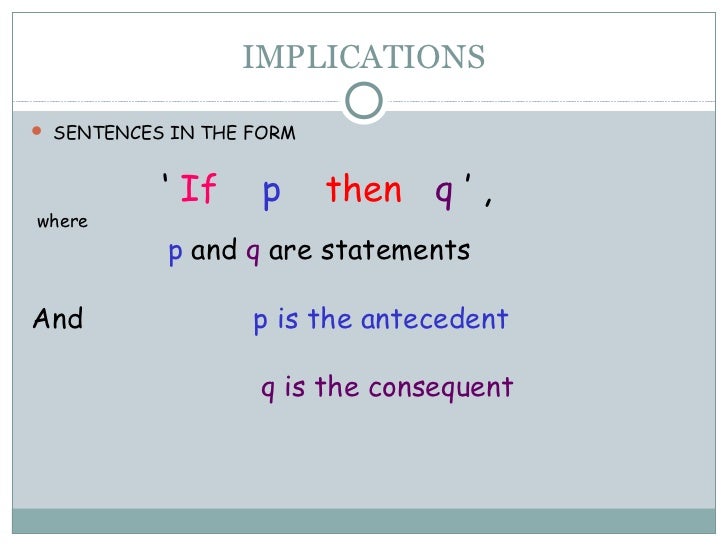
Mathematical Reasoning Form 4 Induction

In the example above notice that 3 is added to the previous term in order to get the current term or current number.

Mathematical reasoning form 4 induction. Mathematically speaking inductive reasoning might take this form. The fibonacci numbers form a famous sequence in mathematics that was investigated by leonardo of pisa 1170. David is a student. Assume it is true for n k.

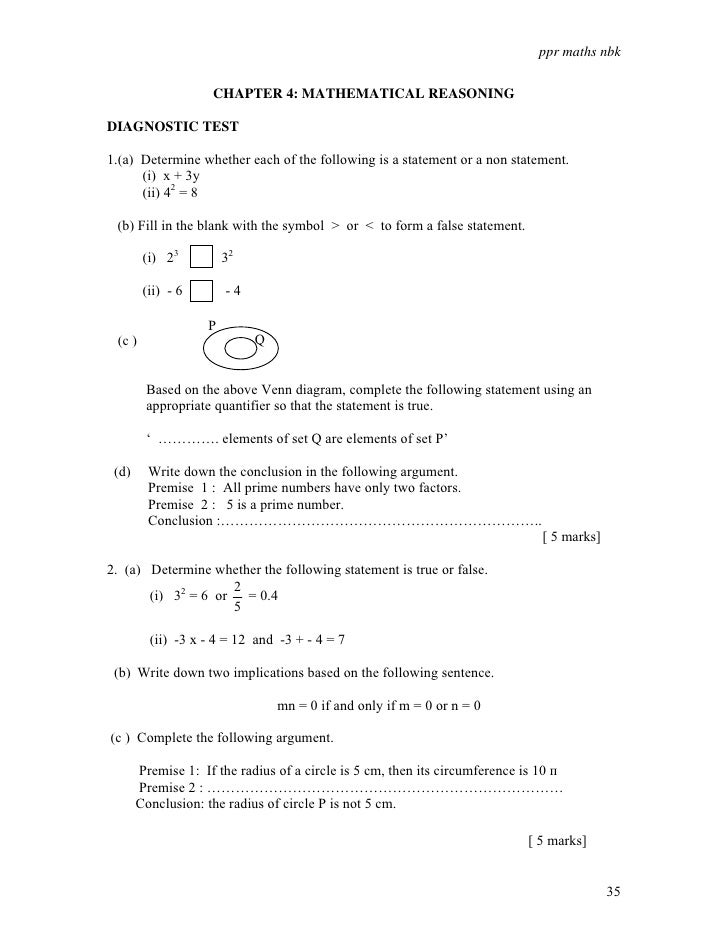
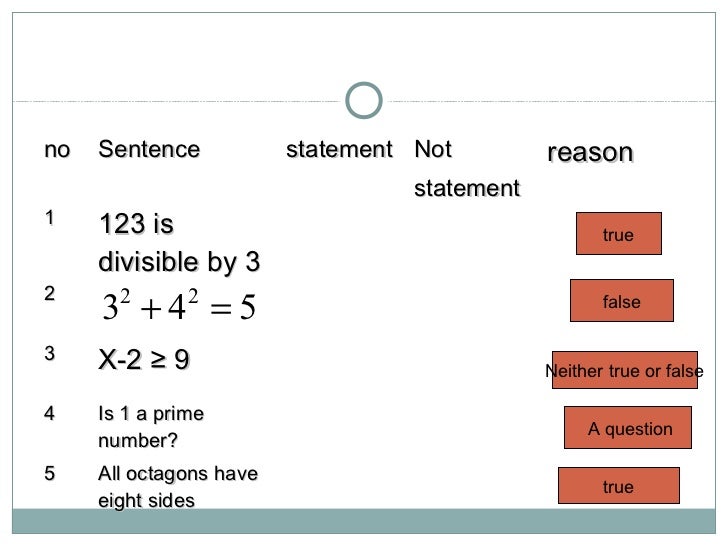
But what is inductive reasoning. Reasoning by deduction and induction1 deduction is the process of making a specific conclusion based on a general statement. Mathematical reasoning diagnostic test 1 a determine whether each of the following is a statement or a non statement. Induction is the process of making a general conclusion based on specific cases.

Making conclusion by deductionthrough reasoning by deduction we can make conclusion for a specific case based on a general statement. One is the process of deduction i e. In a proof by mathematical induction. In section 4 2 we will learn how to extend this method to statements of the form forall n in t p n where t is a certain type of subset of the integers mathbb z.

In this section we will learn a new proof technique called mathematical induction that is often used to prove statements of the form forall n in mathbb n p n. Mathematical induction is an inference rule used in formal proofs and in some form is the foundation of all correctness proofs for computer programs. That is how mathematical induction works. I x 3y ii 42 8 b fill in the blank with the symbol or to form a false statement.

All students in form 4x are present today. Step 1 is usually easy we just have to prove it is true for n 1. Although its name may suggest otherwise mathematical induction should not be confused with inductive reasoning as used in philosophy see problem of induction. Show it is true for first case usually n 1.

The word induction means the method of reasoning about a general statement from the conclusion of. Step 2 show that if it is true for one then it must be true for the. In the world of numbers we say. Inductive reasoning is making conclusions based on patterns you observe the conclusion you reach is called a conjecture.

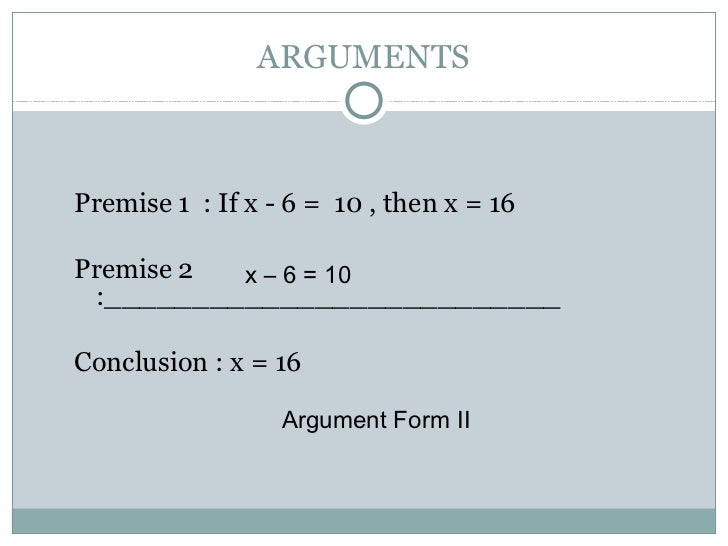
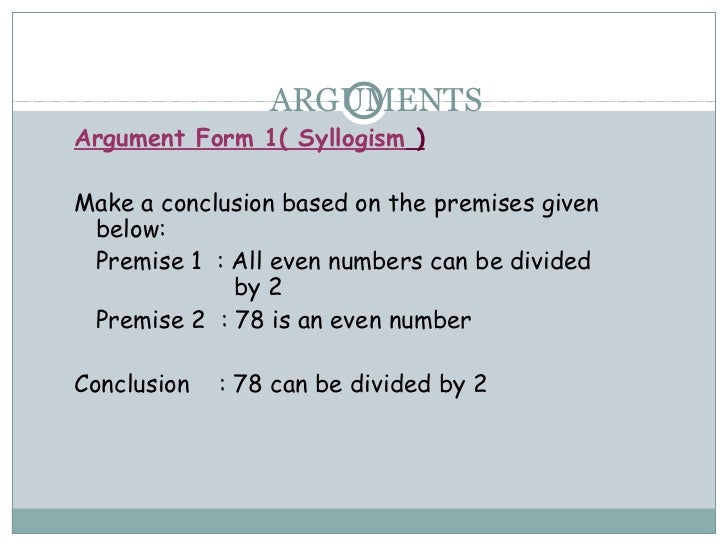
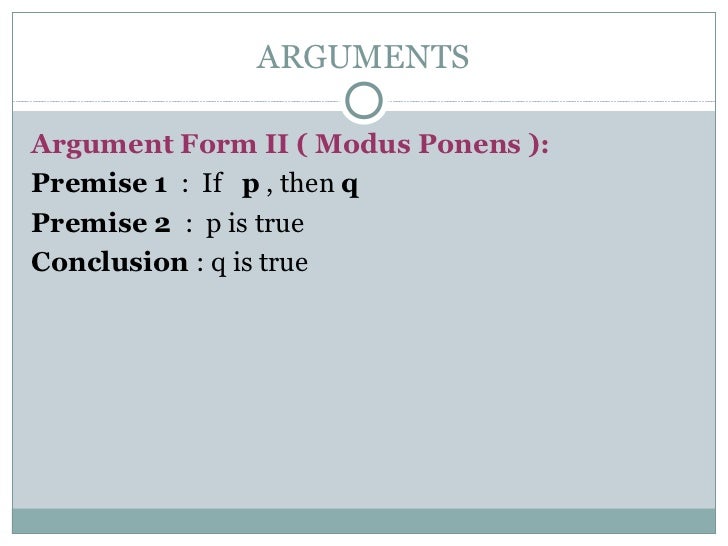
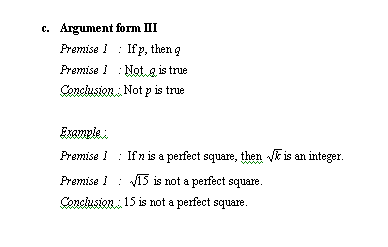
Argument form iii mathematical reasoning deduction and induction reasoning there are two ways of making conclusions through. A deduction b induction deduction. How to do it. Step 1 show that something is true for a specific item.

Mathematical reasoning writing and proof sundstrom 4. In drawing scientific conclusions there are two fundamental processes of reasoning that are commonly employed. Show that if n k is true then n k 1 is also true. The logical conclusion we can make based on this pattern is that to find.

The process of reasoning from general to particular and the other is known as the process of induction which proceeds from particular to general.